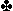Solution to the Overbidder's Challenge

Here are the solutions to the four problems to do with low point-count slams:

1. The 7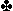 redoubled hand comes from the James Bond novel "Moonraker." Sadly, the hand was omitted from the film version.

2. The minimum number of points needed to make 7NT is eleven. For example, give dummy AQJ to 12 spades, and the ace of hearts, and the person on lead the rest of the major suit cards. Declarer has - - 765432 8765432, which is a poor hand for no trumps.

3. The only distribution of the E-W cards for which you can make 6 (though only due to the inept club lead - surely a heart stands out?!) is as follows:

Game All 10954 2 AQ54 A1032 876 876 KJ 98765       N W        E       S QJ KQJ10 9876 KQJ AK32 A9543 1032 4

Win the lead, ruff a club, finesse a diamond, ruff another club and draw two rounds of trumps. A diamond to the ace now allows the last trump to be drawn and the 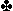10 to be cashed. East is now subjected to an elementary trump squeeze. He can only keep four cards. If he comes down to KQJ and 9 then the 10 is unblocked, before ruffing back to hand to cash the fourth diamond. On the other hand, if he keeps two diamonds and two hearts, declarer crosses to the A, and ruffs the heart out, still having the 10 as an entry to dummy's good heart.

4. By relying on awful defence 7NT can be made with a Yarborough in both hands:

Game All 9753 98765432 9 - AKQJ108642 10 - AKQ       N W        E       S - AKQJ AKQJ108 J109 - - 765432 8765432

West leads the 8 won with the nine, East discarding the A. After another three spades have been cashed, East has parted with his hearts. North now cashes the 9, West carelessly parting with 10, and now the heart suit can be run.